« Indeed, try and do the calculations! »
A correct (AFAICT) first approach is here:
Special Relativity: Inertial Frames
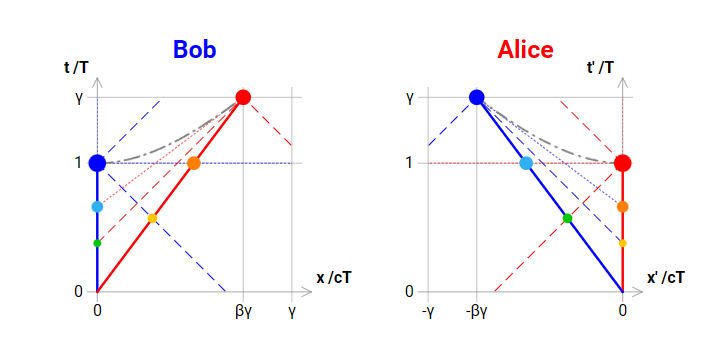
Corollary 1: Retrocausation.
Corollary 2: Nobody understands Relativity.
[UPDATE 2024-12-19]
I have not yet found the time to approach the Twin Paradox proper,
which is about finding coherence conditions for retrocausation...
No comments :
Post a Comment